Everything You Need to Know About Dcimals

We explain what decimals are and how the concept is explained to primary-school children, besides as how they are taught to chronicle decimals to money and measurement, the equivalence between fractions and decimals, sectionalization, rounding and ordering decimals and calculation, subtracting, multiplying and dividing decimals.
What are decimals?
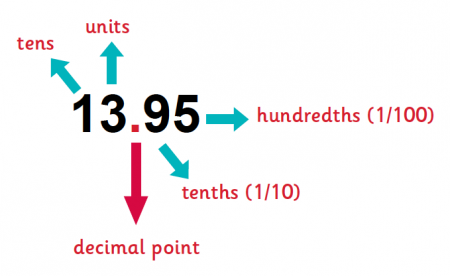
A decimal is a number expressed in the scale of tens. Ordinarily speaking we talk nearly decimals when numbers include a decimal point to correspond a whole number plus a fraction of a whole number (tenths, hundredths, etc.).
A decimal point is a point or dot used to dissever the whole office of a number from the fractional part of a number.

Claim Gratis Decimals Resources Today
- Decimals workbook
- Interactive tutorials
- 100s of worksheets & games
Explaining decimals to children
One of the best means to depict decimals, is to show a kid a blank hundred number square or number chart and explain that this represents 'one':
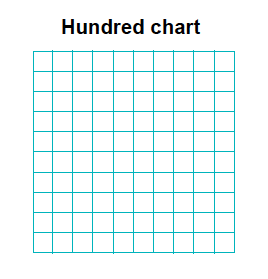
Children need to know that when nosotros talk nearly decimals, it is equally if nosotros were splitting one whole up into smaller parts.
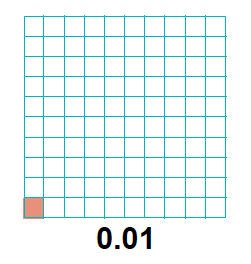
If you coloured in 1 of the squares in the above diagram, this would exist represented as the decimal 0.01 (which is 1 hundredth or 1/100).
If you coloured in x of the squares in the above diagram (therefore colouring in 10/100 or ane/x), this would exist represented by the decimal 0.ane (children may write 0.10 because they have coloured ten squares – you demand to explain that the zeros later on decimal points are always knocked off).

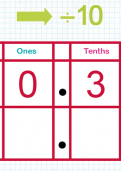
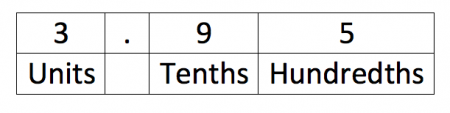
The place value of decimal numbers can be shown the following table:
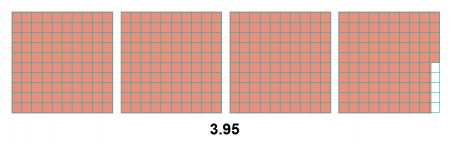
A pictorial representation of this number is:

Learning well-nigh decimals in KS2
In Year 4, children need to empathize the concept of a 'hundredth' and a 'tenth'.
They demand to be able to write decimal equivalents of whatsoever number of tenths and hundredths, for example: 3/10 = 0.3 and 7/100 = 0.07.
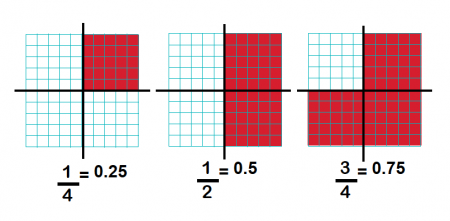
They besides need to know decimal equivalents to 1/iv, 1/two and 3/four. This diagram is a good style of making this concept clear to them:
Children in Twelvemonth iv also need to know the effect of multiplying and dividing numbers ane-digit and two-digit numbers by 10 and 100 (teachers volition talk nigh digits sliding to the left and right).
- 5 multiplied by 10 is l (the five slides one place to the left)
- five divided by x is 0.v (the five slides 1 place to the right)
- five multiplied past 100 is 500 (the five slides 2 places to the left)
- 5 divided by 100 is 0.05 (the 5 slides 2 places to the correct)
- Two-digit number examples: 35 ÷ 10 = three.5, 741 ÷ 100 = 7.41
Partitioning, rounding and ordering decimals
In Twelvemonth 5, children need to count from any given number in decimal steps, so they may be given the following sequence and asked to continue it:
0.3, 0.6, 0.9, ___, ___, ___
(The next decimals would be 1.2, 1.5, 1.eight - it helps to think of counting in 3s here, merely instead of iii, half-dozen, 9, 12, 15, eighteen you lot are using decimal numbers.)
They besides need to sectionalization, round, compare and gild decimals, for instance:
Division eight.49
(eight + 0.iv + 0.09)
Round 7.four to the nearest whole number
(7, because the 4 is smaller than 5, so the number gets rounded downwards)
Round 1.38 to ane decimal identify
(one.iv, because the 3 is rounded upward to 4 because the 8 is bigger than v)
Put these decimals in order: 0.viii, 0.14, i.8, 0.4
(0.fourteen, 0.iv, 0.8, 1.viii)
Compare these decimal numbers: which is larger, 0.nine or 0.iv? Or which is bigger out of 0.12 and 0.82?
(0.ix is larger than 0.iv; 0.82 is larger than 0.12)
They may be asked to position a decimal number on a number line. For case:
Put 6.178 where you think it should go along this number line:

They also need to start calculating with decimals mentally, for example:
- What is half of 3? (one.5)
- What is double 0.34? (0.68)
- What is 0.4 x 5? (two)
- What is 4.v + i.ii? (5.7)
- What is 8 - three.2? (4.8)
- What is 6 divided by 1000? (0.006)
They need to learn efficient written methods for calculation and subtracting decimals with up to two places (for example: 6.29 - 3.84). They need to know and utilise a proficient written method for multiplying a unit of measurement by a decimal (for instance: 4 x 8.iii).
Children demand to become more efficient in quickly converting units of measurement, for example: 140cm = 1.4m, 2800g = 2.8kg, 3100ml = 3.one litres.
In Twelvemonth 5, children demand to continue to exist able to work out equivalent fractions and decimals (for case: knowing that 0.71 = 71/100). They need to learn about thousandths and know that they are represented in the third column after the decimal indicate (0.001 = one thousandth = one/1000),be able to circular decimals with two decimal places to the nearest whole number and to one decimal identify and be able to put a gear up of decimals in guild from smallest to largest. For example:
| 0.9 | 0.19 | 0.half-dozen | 0.78 | 0.412 | 0.52 |
Hither, many children get confused and recall that 0.xix must be bigger than 0.9 (because 19 is bigger than nine). It is a adept idea to write 0.9 equally 0.xc and then explicate that the 0 gets knocked off. You could besides evidence them a blank hundred number foursquare and remind them that with 0.ix, nine tenths of the 100 number square (so nine columns, each with ten squares) need to be coloured in. They may recall that 0.412 is the biggest number, but remind them that considering there are merely 4 tenths, it is smaller than 0.ix, 0.6 and 0.52.
Decimal calculations in Year 6
In Yr 6, children need to starting time multiplying and dividing numbers by 10, 100 and thou (for example: 65 ÷ 100, v ÷ 1000, 4.3 ten 100, etc.). Again, teachers will say that the decimal point stays in the same place, but the numbers move left or correct (depending on whether information technology is multiplication or division). The number of places they movement depends on the number of zeros in the number beingness calculated with.
Children in Year six demand to first multiplying numbers such every bit iii x ane.half dozen or 4 x 5.9. They may do this either using the grid method (where numbers are partitioned and put in a grid), or by curt multiplication (where numbers are put in a cavalcade).
Learning about decimals is a long and involved procedure. It is a difficult concept which children tin can accept a while to go the hang of. This is why laying down crucial groundwork in Year 4 is important: children need to exist given many chances to society decimals and lucifer elementary fractions to decimals (using the blank hundred number square which they tin colour in to help them). This means that they volition properly understand what a decimal is before they tin become onto calculating with them in Twelvemonth 5 and 6.
Source: https://www.theschoolrun.com/what-are-decimals
0 Response to "Everything You Need to Know About Dcimals"
Post a Comment